Today we will learn about the types of signals. Start with the following.
Signals are classified into the following categories:
- Continuous Time and Discrete Time Signals
- Deterministic and Non-deterministic Signals
- Even and Odd Signals
- Periodic and Aperiodic Signals
- Energy and Power Signals
- Real and Imaginary Signals
Continuous Time and Discrete Time Signals
A signal is said to be continuous when it is defined for all instants of time.
A signal is said to be discrete when it is defined at only discrete instants of time/
Deterministic and Non-deterministic Signals
A signal is said to be deterministic if there is no uncertainty with respect to its value at any instant of time. Or, signals which can be defined exactly by a mathematical formula are known as deterministic signals.
A signal is said to be non-deterministic if there is uncertainty with respect to its value at some instant of time. Non-deterministic signals are random in nature hence they are called random signals. Random signals cannot be described by a mathematical equation. They are modelled in probabilistic terms.
Even and Odd Signals
A signal is said to be even when it satisfies the condition x(t) = x(-t)
Example 1: t2, t4… cost etc.
- Let x(t) = t2
x(-t) = (-t)2 = t2 = x(t)
∴,” role=”presentation” style=”box-sizing: border-box; display: inline; line-height: normal; text-align: left; word-spacing: normal; overflow-wrap: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; padding: 0px; margin: 0px; position: relative;”>∴,∴, t2 is even function
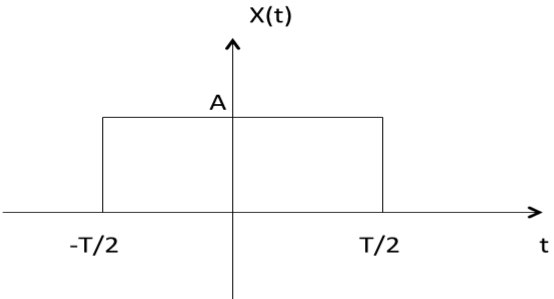
Example 2: As shown in the following diagram, rectangle function x(t) = x(-t) so it is also even function.
A signal is said to be odd when it satisfies the condition x(t) = -x(-t)
Example: t, t3 … And sin t
- Let x(t) = sin t
x(-t) = sin(-t) = -sin t = -x(t)
∴,” role=”presentation” style=”box-sizing: border-box; display: inline; line-height: normal; text-align: left; word-spacing: normal; overflow-wrap: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; padding: 0px; margin: 0px; position: relative;”>∴,∴, sin t is odd function.
Any function ƒ(t) can be expressed as the sum of its even function ƒe(t) and odd function ƒo(t).
- ƒ(t ) = ƒe(t ) + ƒ0(t )
where
ƒe(t ) = ½[ƒ(t ) +ƒ(-t )]
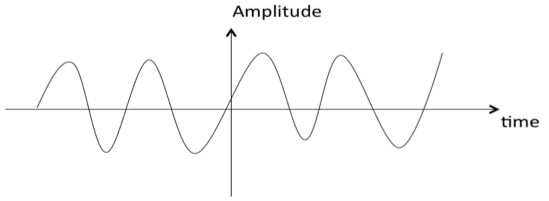
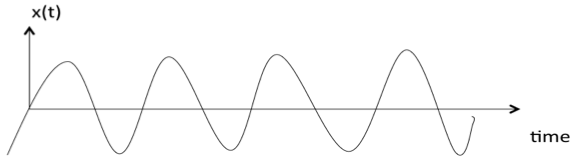
Periodic and Aperiodic Signals
A signal is said to be periodic if it satisfies the condition x(t) = x(t + T) or x(n) = x(n + N).
Where
- T = fundamental time period,
1/T = f = fundamental frequency.
The above signal will repeat for every time interval T0 hence it is periodic with period T0.
Energy and Power Signals
A signal is said to be energy signal when it has finite energy.
A signal is said to be power signal when it has finite power.
NOTE:A signal cannot be both, energy and power simultaneously. Also, a signal may be neither energy nor power signal.
- Power of energy signal = 0
Energy of power signal = ∞
Real and Imaginary Signals
A signal is said to be real when it satisfies the condition x(t) = x*(t)
A signal is said to be odd when it satisfies the condition x(t) = -x*(t)
Example:
- If x(t)= 3 then x*(t)=3*=3 here x(t) is a real signal.
If x(t)= 3j then x*(t)=3j* = -3j = -x(t) hence x(t) is a odd signal.
Note: For a real signal, imaginary part should be zero. Similarly for an imaginary signal, real part should be zero.
The signal is the basis of communication. We provide some signal jammer, which can do scientific research on some specific signals. For details, please see the homepage of this website.